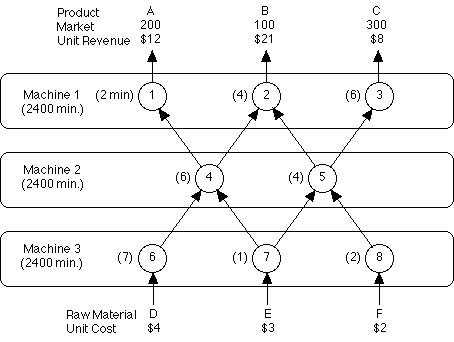
The figure below describes a production process that makes three products, A, B and C. The numbers at the top of the figure provides the maximum sales and unit revenues for the three products. The numbers at the bottom indicate the raw materials used and the unit costs of the raw materials.

The network structure shows the processing requirements for the products. The nodes represent operations the products must pass through and the arcs represent the inputs to the operations. Product A requires one unit from operation 1. Product B requires one unit from operation 2. Product C requires one unit from operation 3.

The product at operation 1 is made from one unit passing out of operation 4. The product at operation 2 is made from one unit each of the products passing out of operations 4 and 5. The product at operation 3 is made from one unit passing out of operation 5.

A unit at operation 4 is made from one unit each from operations 6 and 7. A unit at operation 5 is made from one unit each from operations 7 and 8.

Finally, operation 6 requires one unit of raw material D. Operation 7 requires one unit of raw material E. Operation 8 requires one unit of raw material F.

The numbers adjacent to the nodes are the operation times in minutes. The operations use time on Machine 1, Machine 2 and Machine 3. Each machine has a weekly capacity of 2400 minutes. The products share the capacities of the machines. For example, if 100 units of each product were produced, 1200 minutes would be used on machine 1. Note that product B uses 10 minutes of machine 2 because both operations 4 and 5 are needed for one unit of B.

Use process flow analysis to model this problem. Create three processes, one to model each product. Using the Economic Analysis button, create the Project worksheet. Enter the economic data. Create an LP model with using a button on the Project worksheet. Use the LP model to find the optimum product mix for the three products. Identify the bottleneck machines.