Linearity requires that the revenue for each unit
of product sold remains constant over the range zero to the
value given in Table 1 for maximum sales. In many situations,
however, companies may reduce the unit price of a product to
boost its sales. When such a policy is employed, the product's
marginal revenue decreases with the amount sold.
In economic terms, the marginal revenue is the
derivative of the total revenue function with respect to sales
volume. Call
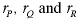
the marginal revenues for the three products, and
assume that each is a linear function of P, Q and R,
respectively. In the following modification, the relevant
objective function coefficients are defined so that the marginal
revenue reduces to half of the original value at maximum sales.
For product P, the original value of revenue is 90/unit and
maximum sales is 100. We model the marginal revenue as 90 -
(45/100)P. The first sale provides a revenue of $90
while the last sale provides a revenue of $45. The marginal
revenues for the three products are decreasing linear functions
of production volume.
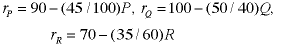
The total revenue associated with
the products is the integral of the marginal values. With
a linear marginal revenue, the total revenue is a concave quadratic
function.
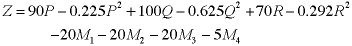
This is called a quadratic, separable function because the
highest order of the nonlinear terms is 2, and each term is
a function of only one variable. We substitute this objective
in the LP to form a nonlinear programming model. The linear
constraints of the LP are still valid.

