When several alternatives are being considered, each will
have different cash flows over time. We assign the notation
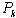 as the investment and
as the investment and  and
and  to indicate the income and the cost for alternative k
at time t.
to indicate the income and the cost for alternative k
at time t. 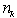 is
the life of alternative k and K is the number
of alternatives. In the typical problem, the alternatives
are mutually exclusive; only one may be chosen.
is
the life of alternative k and K is the number
of alternatives. In the typical problem, the alternatives
are mutually exclusive; only one may be chosen.
Alternatives may be compared with the present worth method
or the annual worth method. For the present worth method,
the NPW of the cash flows for alternative k is computed
using the MARR.

We use the argument 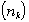 to
emphasize that the present worth is computed over
to
emphasize that the present worth is computed over 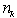 periods.
periods.
When all alternatives have the same life, the
one with the greatest NPW is selected as the best.
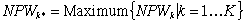
When the alternatives have different lives, we must compare
their present worths over a common time horizon called the
study period. There are two ways to establish a study
period. The simplest is to truncate the longer lived alternatives
at the termination of the alternative with the smallest life.
We must then include a salvage value for the truncated alternatives
for the study period. The second method is to use
a study period equal to the least common multiple
of the lives. We use the latter method because it does not
require estimates of the salvage values for time periods other
than the project life. When we have two or more alternatives
let n be the least common multiple of the lives.
One way to calculate the NPW over the study period is to
first compute the NAW of each alternative and then compute
the present worth for n periods.
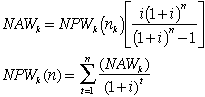
The best alternative is the one with the greatest
value of 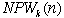 .
An equivalent and sometimes easier method is to chose the
alternative with the greatest value of
.
An equivalent and sometimes easier method is to chose the
alternative with the greatest value of 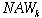 .
.
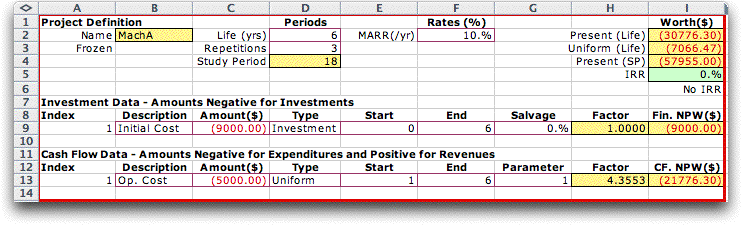
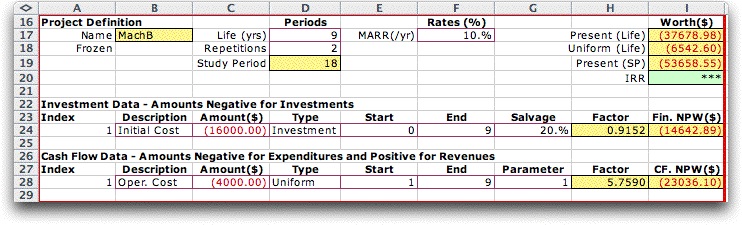
These concepts are illustrated with the two
alternatives below. The least common multiple of the two
lives (6 and 9) is 18 years. Comparing the two on the basis
of a single life using the NPW values in I2 and I17 respectively
indicates that Machine A should be selected, but this is
incorrect. Comparing their NAW values in cells I3 and I18
we see that Machine B should be selected. The NPW values
over the study period in cells I4 and I19 also indicate
that Machine B is best. This is the correct decision.