Many organizations deliver their services via branches. A good example is a bank. Usually there is a main bank and a number of branch banks. The branches all deliver the same set of services (called outputs) and use the same set of resources (called inputs). Other examples are primary schools in a school system, school systems with in a state, state and federal programs with local offices, and large grocers with individual stores. This section will call the individual office a decision making unit (DMU).

Managers of the organization are interested in evaluating offices relative to each other. Such information has many uses such as allocating budgets for resources, identifying the worst offices with the possibility of improvement or disinvestment, and identifying the offices with the "best" practices that might be passed on to those performing at a lower level.

The small example used in this section is from lecture notes by J. E. Beasley. A bank has four branches. Two output measures are specified, personal transactions and business transactions. The number of staff members for a branch is the single input measure. The quantities for these three data items for a single year are in the table below. The outputs are thousands of units.

An individual branch is called a decision making unit or DMU. The Chief Financial Officer (CFO) of the bank wants to compare the four branches or DMU's.

One way to compare the branches is to add together the two output measures to obtain a single measure. The table below shows the combined outputs with the title transactions. The number of transactions is simply the sum of the personal and business transactions shown in the data table.

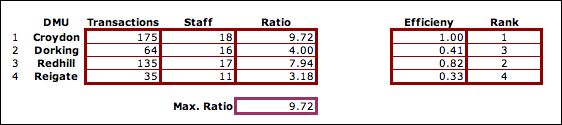
With only one output and one input we can compare DMU's by computing the ratio: output/input or transactions per staff member. The Ratio column is obtained by dividing the transactions number by the staff number. This suggests that Croydon is the best branch because its ratio is larger than all the rest.

For convenience we want a measure that lies between 0 and 1, so we divide the ratios by the maximum ratio (9.72). The results for the four branches are in the column above labeled Efficiency. Efficiency is a good word for the measure since it divides output by input and is similar to comparisons made in other fields. For instance one can speak of the efficiency of a solar cell by dividing the electrical power produced in the cell by the light power falling on the cell. Both input and output power are measured in watts. The quantities are difficult to measure, but efficiency compares the result to a standard to obtain a meaningful value. For instance the record for solar cell efficiency is 42%. One would expect that solar cell efficiency will never approach 1.

The efficiency number computed in the table above is not the same. The value of 1 does not mean Croydon is perfectly translating inputs to outputs. It only means that Croydon has the best output over input ratio of the four banks. If one accepts the measure it can be used to rank the four DMU's. The result is shown in the rank column. The CFO has the result that she needs. She brings her ranking to a meeting with the branch managers.

The manager of the Croydon branch is pleased, but the other branch managers call the ranking unfair. They say the measure used to obtain the ranking is flawed. Simply adding the outputs may not be correct. Different efficiencies would be obtained if a different weight were applied to each measure. One could say that the personal transactions were not as important as the business transactions. Someone could propose that each business transition be given three times the weight of a personal transaction. This would yield different efficiency values and perhaps a different ranking.

The CFO does not want to pursue a policy that alienates the managers. No one, except the Croydon bank manager will accept the efficiency as calculated above to compute the ranking. If the branch managers accept the idea that some ratio of outputs to inputs can be used for comparison, the problem is to agree on the best weights for the output factors. Of course, the branch managers will not agree on the appropriate weights.

The operations analyst attending the meeting suggests to the CFO that the DEA method might help in seeking agreement. After a private discussion with the analyst, the CFO announces that the managers may independently weigh the factors any way they like. The CFO adds that the managers must each use the input and output data (from the tables above) and evaluate the efficiencies for all four branches with the same set of weights. Each branch must work without interacting with any other branch. The managers agree to the restrictions and agree to meet in one week to provide their answers.

DEA is a method to deal with situations like this. Although it does not solve the ranking problem directly, the DEA provides some objective measures to obtain a partial ranking. The DEA add-in embodies the computational tools necessary to implement the method.The following pages of this section describe the method along with the add-in features. Only the basics of DEA are presented. There are many papers and books on the subject as reported in a web site by Emrouznejad. Several commercial programs provide more detailed analysis than this add-in. There are many practical questions involved with actual implementations that are not considered here.